Wikipedia

Template:Regular hyperbolic tiling table/sandbox

< Template:Regular hyperbolic tiling table
Regular hyperbolic tiling table
Spherical (improper/Platonic)/Euclidean/hyperbolic (Poincaré disc: compact/paracompact/noncompact) tessellations with their Schläfli symbol
p \ q 1 2 3 4 5 6 7 8 ... ∞ ... iπ/λ
1
{1,1}
 
{1,2}
 

 {1,3}
 

 {1,4}
 

 {1,5}
 

 {1,6}
 

 {1,7}
 

 {1,8}
 

 {1,∞}
 

 {1,iπ/λ}
 
2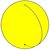
{2,1}
 
{2,2}
{2,3}
{2,4}
{2,5}
{2,6}
{2,7}
{2,8}
{2,∞}
{2,iπ/λ}
3
{3,1}
 
{3,2}
{3,3}
{3,4}
{3,5}
{3,6}
{3,7}
{3,8}
{3,∞}
{3,iπ/λ}
4
{4,1}
 
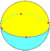{4,2}
{4,3}
{4,4}
{4,5}
{4,6}
{4,7}
{4,8}
{4,∞}
{4,iπ/λ}
5
{5,1}
 
{5,2}
{5,3}
{5,4}
{5,5}
{5,6}
{5,7}
{5,8}
{5,∞}
{5,iπ/λ}
6 {6,1}
 
{6,2}
{6,3}
{6,4}
{6,5}
{6,6}
{6,7}
{6,8}
{6,∞}
{6,iπ/λ}
7 {7,1}
  		{7,2}
{7,3}
{7,4}
{7,5}
{7,6}
{7,7}
{7,8}
{7,∞}
 {7,iπ/λ}
8 {8,1}
  		{8,2}
{8,3}
{8,4}
{8,5}
{8,6}
{8,7}
{8,8}
{8,∞}
 {8,iπ/λ}
...
∞ {∞,1}
 
{∞,2}
{∞,3}
{∞,4}
{∞,5}
{∞,6}
{∞,7}
{∞,8}
{∞,∞}
{∞,iπ/λ}
...
iπ/λ {iπ/λ,1}
 
{iπ/λ,2}
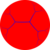{iπ/λ,3}
{iπ/λ,4}
{iπ/λ,5}
{iπ/λ,6}
 {iπ/λ,7}
 {iπ/λ,8}
{iπ/λ,∞}
{iπ/λ,iπ/λ}
Retrieved from "https://en.wikipedia.org/w/index.php?title=Template:Regular_hyperbolic_tiling_table/sandbox&oldid=1252429150"