Wikipedia

Auxetics

Auxetic metamaterials are a type of metamaterial with a negative Poisson's ratio, so that axial elongation causes transversal elongation (in contrast to an ordinary material, where stretching in one direction causes compression in the other direction).

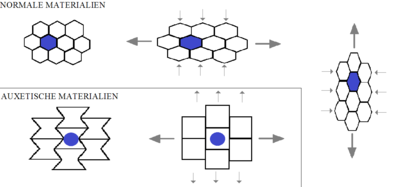
Auxetics can be single molecules, crystals, or a particular structure of macroscopic matter.[1][2]

Auxetic materials are used in protective equipment such as body armor, helmets, and knee pads, as they absorb energy more effectively than traditional materials.[3][4] They are also used in devices such as medical stents or implants. Auxetic fabrics can be used to create comfortable and flexible clothing, as well as technical fabrics for applications such as aerospace and sports equipment.[5] Auxetic materials can also be used to create acoustic metamaterials for controlling sound and vibration.[6]

History

edit

The term auxetic derives from the Greek word auxetikos (αὐξητικός) which means 'that which tends to increase' and has its root in the word auxesis (αὔξησις), meaning 'increase' (noun). This terminology was coined by Professor Ken Evans of the University of Exeter.[7][2] One of the first artificially produced auxetic materials, the RFS structure (diamond-fold structure), was invented in 1978 by the Berlin researcher K. Pietsch. Although he did not use the term auxetics, he describes for the first time the underlying lever mechanism and its non-linear mechanical reaction so he is therefore considered the inventor of the auxetic net. The earliest published example of a material with negative Poisson's constant is due to A. G. Kolpakov in 1985, "Determination of the average characteristics of elastic frameworks"; the next synthetic auxetic material was described in Science in 1987, entitled "Foam structures with a Negative Poisson's Ratio"[1] by R.S. Lakes from the University of Wisconsin Madison. The use of the word auxetic to refer to this property probably began in 1991.[8] Recently, cells were shown to display a biological version of auxeticity under certain conditions. [9]

Designs of composites with inverted hexagonal periodicity cell (auxetic hexagon), possessing negative Poisson ratios, were published in 1985.[10]

For these reasons, gradually, many researchers have become interested in the unique properties of Auxetics. This phenomenon is visible in the number of publications (Scopus search engine), as shown in the following figure. In 1991, there was only one publication. However, in 2016, around 165 publications were released, so the number of publications has exploded - a 165-fold increase in just 25 years - clearly showing that the topic of Auxetics is drawing considerable attention.[11] However, although Auxetics are promising structures and have a lot of potential in science and engineering, their widespread application in multiple fields is still a challenge. Therefore, additional research related to Auxetics is required for widespread applications.

Properties

edit

Typically, auxetic materials have low density, which is what allows the hinge-like areas of the auxetic microstructures to flex.[12]

At the macroscale, auxetic behaviour can be illustrated with an inelastic string wound around an elastic cord. When the ends of the structure are pulled apart, the inelastic string straightens while the elastic cord stretches and winds around it, increasing the structure's effective volume. Auxetic behaviour at the macroscale can also be employed for the development of products with enhanced characteristics such as footwear based on the auxetic rotating triangles structures developed by Grima and Evans[13][14][15] and prosthetic feet with human-like toe joint properties.[16]

Auxetic materials also occur organically, although they are structurally different from man-made metamaterials. For example, the nuclei of mouse embryonic stem cells in a transition state display auxetic behavior.[17]

Examples

edit
 
In footwear, auxetic design allows the sole to expand in size while walking or running, thereby increasing flexibility.

Examples of auxetic materials include:

  • Auxetic polyurethane foam[18][19]
  • Nuclei of mouse embryonic stem cells in exiting pluripotent state [17]
  • α-Cristobalite.[20]
  • Certain states of crystalline materials: Li, Na, K, Cu, Rb, Ag, Fe, Ni, Co, Cs, Au, Be, Ca, Zn, Sr, Sb, MoS2, BAsO4, and others.[21][22][23]
  • Certain rocks and minerals[24]
  • Graphene, which can be made auxetic through the introduction of vacancy defects[25][26]
  • Carbon diamond-like phases[27]
  • Two-dimensional tungsten semicarbide[28]
  • Noncarbon nanotubes[29][30]
  • Living bone tissue (although this is only suspected)[24]
  • Tendons within their normal range of motion.[31]
  • Specific variants of polytetrafluorethylene polymers such as Gore-Tex[32]
  • Several types of origami folds like the Diamond-Folding-Structure (RFS), the herringbone-fold-structure (FFS) or the miura fold,[33][34] and other periodic patterns derived from it.[35][36]
 
Production of auxetic metamaterials through the introduction of patterned microstructural cuts using direct laser cutting. The thin rubber surface with perforated architecture covers a spherical surface (orange)[37]
  • Tailored structures designed to exhibit special designed Poisson's ratios.[38][39][40][41][42][43]
  • Chain organic molecules. Recent researches revealed that organic crystals like n-paraffins and similar to them may demonstrate an auxetic behavior.[44]

See also

edit

References

edit
  1. ^ a b Lakes, R.S. (27 February 1987), "Foam structures with a negative Poisson's ratio", Science, 235 (4792): 1038–40, Bibcode:1987Sci...235.1038L, doi:10.1126/science.235.4792.1038, PMID 17782252, S2CID 21386778.
  2. ^ a b Evans, Ken (1991), "Auxetic polymers: a new range of materials.", Endeavour, 15 (4): 170–174, doi:10.1016/0160-9327(91)90123-S.
  3. ^ Haid, Daniel; Foster, Leon; Hart, John; Greenwald, Richard; Allen, Tom; Sareh, Pooya; Duncan, Olly (1 November 2023). "Mechanical metamaterials for sports helmets: structural mechanics, design optimisation, and performance". Smart Materials and Structures. 32 (11): 113001. doi:10.1088/1361-665X/acfddf.
  4. ^ Duncan, Olly; Shepherd, Todd; Moroney, Charlotte; Foster, Leon; Venkatraman, Praburaj D.; Winwood, Keith; Allen, Tom; Alderson, Andrew (6 June 2018). "Review of Auxetic Materials for Sports Applications: Expanding Options in Comfort and Protection". Applied Sciences. 8 (6): 941. doi:10.3390/app8060941.
  5. ^ Duncan, Olly; Shepherd, Todd; Moroney, Charlotte; Foster, Leon; Venkatraman, Praburaj D.; Winwood, Keith; Allen, Tom; Alderson, Andrew (6 June 2018). "Review of Auxetic Materials for Sports Applications: Expanding Options in Comfort and Protection". Applied Sciences. 8 (6): 941. doi:10.3390/app8060941.
  6. ^ Liu, Yangzuo; Zhao, Changfang; Xu, Cheng; Ren, Jie; Zhong, Jianlin (1 December 2023). "Auxetic meta-materials and their engineering applications: a review". Engineering Research Express. 5 (4): 042003. doi:10.1088/2631-8695/ad0eb1. ISSN 2631-8695.
  7. ^ Quinion, Michael (9 November 1996), Auxetic.
  8. ^ Evans, Ken (1991), "Auxetic polymers: a new range of materials", Endeavour, 15 (4): 170–174, doi:10.1016/0160-9327(91)90123-S.
  9. ^ Morrish, RB (2019), "Single Cell Imaging of Nuclear Architecture Changes", Front. Cell Dev. Biol., 7: 141, doi:10.3389/fcell.2019.00141, PMC 6668442, PMID 31396512.
  10. ^ Kolpakov, A.G. (1985). "Determination of the average characteristics of elastic frameworks". Journal of Applied Mathematics and Mechanics. 49 (6): 739–745. Bibcode:1985JApMM..49..739K. doi:10.1016/0021-8928(85)90011-5.
  11. ^ Ren, Xin, et al. "Auxetic metamaterials and structures: a review." Smart materials and structures 27.2 (2018): 023001.
  12. ^ A stretch of the imagination – 7 June 1997 – New Scientist Space
  13. ^ Grima, JN; Evans, KE (2000). "Auxetic behavior from rotating squares". Journal of Materials Science Letters. 19 (17): 1563–1565. doi:10.1023/A:1006781224002. S2CID 138455050.
  14. ^ Grima, JN; Evans, KE (2006). "Auxetic behavior from rotating triangles". Journal of Materials Science. 41 (10): 3193–3196. Bibcode:2006JMatS..41.3193G. doi:10.1007/s10853-006-6339-8. S2CID 137547536.
  15. ^ "Nike Free 2016 product press release".
  16. ^ Hong, Woolim; Kumar, Namita Anil; Patrick, Shawanee; Um, Hui-Jin; Kim, Heon-Su; Kim, Hak-Sung; Hur, Pilwon (2022). "Empirical Validation of an Auxetic Structured Foot With the Powered Transfemoral Prosthesis". IEEE Robotics and Automation Letters. 7 (4): 11228–11235. doi:10.1109/LRA.2022.3194673. ISSN 2377-3766. S2CID 251170703.
  17. ^ a b Tripathi, Kamal; Menon, Gautam I. (28 October 2019). "Chromatin Compaction, Auxeticity, and the Epigenetic Landscape of Stem Cells". Physical Review X. 9 (4): 041020. doi:10.1103/PhysRevX.9.041020. S2CID 209958957.
  18. ^ Li, Yan; Zeng, Changchun (2016). "On the successful fabrication of auxetic polyurethane foams: Materials requirement, processing strategy and conversion mechanism". Polymer. 87: 98–107. doi:10.1016/j.polymer.2016.01.076.
  19. ^ Li, Yan; Zeng, Changchun (2016). "Room-Temperature, Near-Instantaneous Fabrication of Auxetic Materials with Constant Poisson's Ratio over Large Deformation". Advanced Materials. 28 (14): 2822–2826. doi:10.1002/adma.201505650. PMID 26861805. S2CID 5260896.
  20. ^ Yeganeh-Haeri, Amir; Weidner, Donald J.; Parise, John B. (31 July 1992). "Elasticity of α-Cristobalite: A Silicon Dioxide with a Negative Poisson's Ratio". Science. 257 (5070): 650–652. Bibcode:1992Sci...257..650Y. doi:10.1126/science.257.5070.650. ISSN 0036-8075. PMID 17740733. S2CID 137416819.
  21. ^ Goldstein, R.V.; Gorodtsov, V.A.; Lisovenko, D.S. (2013). "Classification of cubic auxetics". Physica Status Solidi B. 250 (10): 2038–2043. doi:10.1002/pssb.201384233. S2CID 117802510.
  22. ^ Gorodtsov, V.A.; Lisovenko, D.S. (2019). "Extreme values of Young's modulus and Poisson's ratio of hexagonal crystals". Mechanics of Materials. 134: 1–8. doi:10.1016/j.mechmat.2019.03.017. S2CID 140493258.
  23. ^ Grima-Cornish, JN; Vella-Zarb, L; Grima, JN (2020). "Negative Linear Compressibility and Auxeticity in Boron Arsenate". Annalen der Physik. 532 (5): 1900550. Bibcode:2020AnP...53200550G. doi:10.1002/andp.201900550. S2CID 216414513.
  24. ^ a b Burke, Maria (7 June 1997), "A stretch of the imagination", New Scientist, 154 (2085): 36
  25. ^ Grima, J. N.; Winczewski, S.; Mizzi, L.; Grech, M. C.; Cauchi, R.; Gatt, R.; Attard, D.; Wojciechowski, K.W.; Rybicki, J. (2014). "Tailoring Graphene to Achieve Negative Poisson's Ratio Properties". Advanced Materials. 27 (8): 1455–1459. doi:10.1002/adma.201404106. PMID 25504060. S2CID 19738771.
  26. ^ Grima, Joseph N.; Grech, Michael C.; Grima-Cornish, James N.; Gatt, Ruben; Attard, Daphne (2018). "Giant Auxetic Behaviour in Engineered Graphene". Annalen der Physik. 530 (6): 1700330. Bibcode:2018AnP...53000330G. doi:10.1002/andp.201700330. ISSN 1521-3889. S2CID 125889091.
  27. ^ Rysaeva, L.Kh.; Baimova, J.A.; Lisovenko, D.S.; Gorodtsov, V.A.; Dmitriev, S.V. (2019). "Elastic properties of fullerites and diamond-like phases". Physica Status Solidi B. 256 (1): 1800049. Bibcode:2019PSSBR.25600049R. doi:10.1002/pssb.201800049.
  28. ^ Stocek, N.B.; Ullah, F.; Fanchini, G. (2024). "Giant auxetic behavior in remote-plasma synthesized few-layer tungsten semicarbide". Materials Horizons. 11: 3066–3075. doi:10.1039/D3MH02193A.
  29. ^ Goldstein, R.V.; Gorodtsov, V.A.; Lisovenko, D.S.; Volkov, M.A. (2014). "Negative Poisson's ratio for cubic crystals and nano/microtubes". Physical Mesomechanics. 17 (2): 97–115. doi:10.1134/S1029959914020027. S2CID 137267947.
  30. ^ Bryukhanov, I.A.; Gorodtsov, V.A.; Lisovenko, D.S. (2019). "Chiral Fe nanotubes with both negative Poisson's ratio and Poynting's effect. Atomistic simulation". Journal of Physics: Condensed Matter. 31 (47): 475304. Bibcode:2019JPCM...31U5304B. doi:10.1088/1361-648X/ab3a04. PMID 31398716. S2CID 199519252.
  31. ^ Gatt R, Vella Wood M, Gatt A, Zarb F, Formosa C, Azzopardi KM, Casha A, Agius TP, Schembri-Wismayer P, Attard L, Chockalingam N, Grima JN (2015). "Negative Poisson's ratios in tendons: An unexpected mechanical response" (PDF). Acta Biomater. 24: 201–208. doi:10.1016/j.actbio.2015.06.018. PMID 26102335.
  32. ^ Auxetic materials, 9 March 2001.
  33. ^ Mark, Schenk (2011). Folded Shell Structures, PhD Thesis (PDF). University of Cambridge, Clare College.
  34. ^ Lv, Cheng; Krishnaraju, Deepakshyam; Konjevod, Goran; Yu, Hongyu; Jiang, Hanqing (2015). "Origami based Mechanical Metamaterials". Scientific Reports. 4: 5979. doi:10.1038/srep05979. PMC 4124469. PMID 25099402.
  35. ^ Eidini, Maryam; Paulino, Glaucio H. (2015). "Unraveling metamaterial properties in zigzag-base folded sheets". Science Advances. 1 (8): e1500224. arXiv:1502.05977. Bibcode:2015SciA....1E0224E. doi:10.1126/sciadv.1500224. ISSN 2375-2548. PMC 4643767. PMID 26601253.
  36. ^ Eidini, Maryam (2016). "Zigzag-base folded sheet cellular mechanical metamaterials". Extreme Mechanics Letters. 6: 96–102. arXiv:1509.08104. doi:10.1016/j.eml.2015.12.006. S2CID 118424595.
  37. ^ Mizzi, Luke; Salvati, Enrico; Spaggiari, Andrea; Tan, Jin-Chong; Korsunsky, Alexander M. (2020). "Highly stretchable two-dimensional auxetic metamaterial sheets fabricated via direct-laser cutting". International Journal of Mechanical Sciences. 167: 105242. doi:10.1016/j.ijmecsci.2019.105242. hdl:11380/1185053. ISSN 0020-7403. S2CID 210231091.
  38. ^ Tiemo Bückmann; et al. (May 2012). "Tailored 3D Mechanical Metamaterials Made by Dip-in Direct-Laser-Writing Optical Lithography". Advanced Materials. 24 (20): 2710–2714. doi:10.1002/adma.201200584. PMID 22495906. S2CID 205244958.
  39. ^ Grima-Cornish, James N.; Grima, Joseph N.; Evans, Kenneth E. (2017). "On the Structural and Mechanical Properties of Poly(Phenylacetylene) Truss-Like Hexagonal Hierarchical Nanonetworks". Physica Status Solidi B. 254 (12): 1700190. Bibcode:2017PSSBR.25400190G. doi:10.1002/pssb.201700190. hdl:10871/31485. ISSN 1521-3951. S2CID 126184802.
  40. ^ Cabras, Luigi; Brun, Michele (2014). "Auxetic two-dimensional lattices with Poisson's ratio arbitrarily close to −1". Proceedings of the Royal Society A: Mathematical, Physical and Engineering Sciences. 470 (2172): 20140538. arXiv:1407.5679. Bibcode:2014RSPSA.47040538C. doi:10.1098/rspa.2014.0538. ISSN 1364-5021.
  41. ^ Carta, Giorgio; Brun, Michele; Baldi, Antonio (2016). "Design of a porous material with isotropic negative Poisson's ratio". Mechanics of Materials. 97: 67–75. doi:10.1016/j.mechmat.2016.02.012.
  42. ^ Cabras, Luigi; Brun, Michele (2016). "A class of auxetic three-dimensional lattices". Journal of the Mechanics and Physics of Solids. 91: 56–72. arXiv:1506.04919. Bibcode:2016JMPSo..91...56C. doi:10.1016/j.jmps.2016.02.010. S2CID 85547530.
  43. ^ Kaminakis, N; Stavroulakis, G (2012). "Topology optimization for compliant mechanisms, using evolutionary-hybrid algorithms and application to the design of auxetic materials". Composites Part B Engineering. 43 (6): 2655–2668. doi:10.1016/j.compositesb.2012.03.018.
  44. ^ Stetsenko, M (2015). "Determining the elastic constants of hydrocarbons of heavy oil products using molecular dynamics simulation approach". Journal of Petroleum Science and Engineering. 126: 124–130. doi:10.1016/j.petrol.2014.12.021.
edit
Retrieved from "https://en.wikipedia.org/w/index.php?title=Auxetics&oldid=1253388933"